In spatially structured populations, simple deterministic update rules can give rise to emergent, complex spatio-temporal patterns. On the population level, deterministic updating requires that all individuals are simultaneously updated. This reflects an annual reproductive cycle and contrasts with asynchronous updating, which approximates a continuous time system. On the individuals' level, the updating of strategies needs to be deterministic too. One particularly simple rule is to adopt the strategy of the best performing neighbor - and in case there is a tie, keep your own strategy. (Note that for more than two strategies, a tie may occur between two different strategies. To resolve this 'degeneracy', it is usually sufficient to choose slightly different parameters or otherwise an additional rule is required to specify which strategy is adopted. This happens, for example, in the rock-scissors-paper game or the voluntary public goods game). For symmetrical initial configurations, the symmetry is preserved by the deterministic updating, which gives rise to fascinating dynamic patterns ressembling evolutionary kaleidoscopes. Admittedly, the scientific relevance of these patterns is rather limited because dynamics is prone to disturbances but do have quite some entertainment value.

Similar evolutionary kaleidoscopes can be found in 2×2 games with the prisoner's dilemma and snowdrift game as prominent representatives. Interestingly, the traditional formulation of the public goods game does not produce such dynamic patterns, except in the case of voluntary participation, which includes a third strategic type, the loners. In the synergy and discounting framework, however, both public goods type interactions as well as snowdrift type interactions readily produce appealing dynamical patterns.

Scenarios

Clicking either on one of the pictures below or the corresponding link to the right will open a new window with a running applet illustrating the respective scenario. You can use this as a starting point to study effects of variations of the parameters.

Legend

Evolution of cooperators and defectors in well-mixed populations where individuals interacting in social dilemmas with discounted or synergistically enhanced accumulation of cooperative benefits.

Color code:CooperatorsDefectors
 New cooperatorsNew defectors
-

Public goods type interactions - Moore neighborhood

The Moore neighborhood limits interactions of an individual to its eight neighbors reachable by a chess-kings-move and thus limits public goods interactions to groups of at most N = 9. In public goods type interactions defection is dominant in well-mixed populations but the ability to form clusters enables cooperators to survive in spatially structured populations.

The rectangular lattice is initialized with a single defector in a sea of cooperators. Note that arbitrary initial configurations can be generated by altering the strategies of any lattice site with a mouse click (this only works if no simulation is running). The snapshot on the left was taken after 1089 generations.

-

Public goods type interactions - von Neumann neighborhood

The von Neumann neighborhood includes the four nearest neighbors to the east, north, west and south and thus the interaction group size is limited a maximum of N = 5.

The lattice is again initialized with a single defector in a sea of cooperators. As above, any initial configuration can be generated with mouse clicks on the lattice. The snapshot on the left was taken after 224 generations. After a fulminant struggle, cooperators disappear after 326 generations.

-

Snowdrift type interactions - Moore neighborhood

In snowdrift type interactions, cooperators and defectors co-exist in well-mixed populations. Interestingly, spatial structure can promote cooperation just as in public goods type interactions but it can also inhibit or even eliminate cooperation. But in any case this produces further fascinating and ever changing spatio-temporal patterns. With the Moore neighborhood, the maximum group size is N = 9.

As before, the lattice is initialized with a single defector in a sea of cooperators. The snapshot on the left was taken after 241 generations.

-

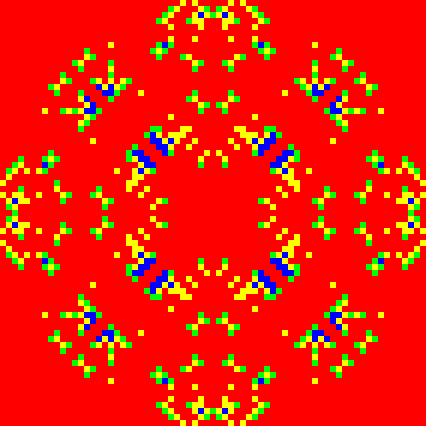Snowdrift type interactions - von Neumann neighborhood

Snowdrift type interactions on a lattice with von Neumann neighborhood, i.e. for interaction groups up to N = 5 individuals. Cooperators persist at very low frequencies and are constantly on the brink of extinction.

The lattice is again initialized with a single defector in a sea of cooperators. The snapshot on the left was taken after 232 generations.

-

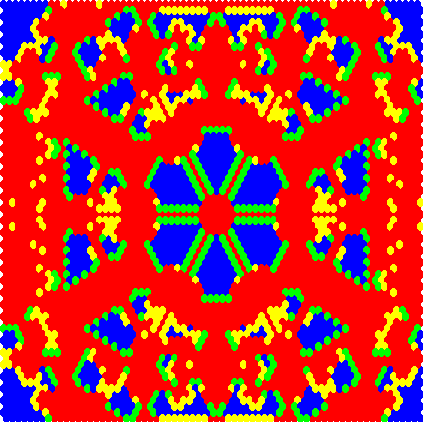Snowdrift type interactions - hexagonal lattice

On a hexagonal lattice, each player interacts with its six nearest neighbors which limits the group size to a maximum of N = 7.

The hexagonal lattice is initialized with a single defector in a sea of cooperators. Mouse clicks again toggle the strategy of any lattice site (provided that no simulation is running) in order to generate arbitrary initial configurations. The snapshot on the left was taken after 48 generations.

 

Triangular lattices

Interestingly, similar evolutionary kaleidoscopes have not yet been observed for triangular lattices. Roughly speaking, such dynamical patterns require that clusters of cooperators grow along edges and shrink at corners. The distinction between edges and corners is obvious on rectangular lattices but is less clear on hexagonal and triangular lattices. A careful analysis is likely to reveal that triangular lattices prevent the emergence of kaleidoscopes - please let me know if you find a counter example!